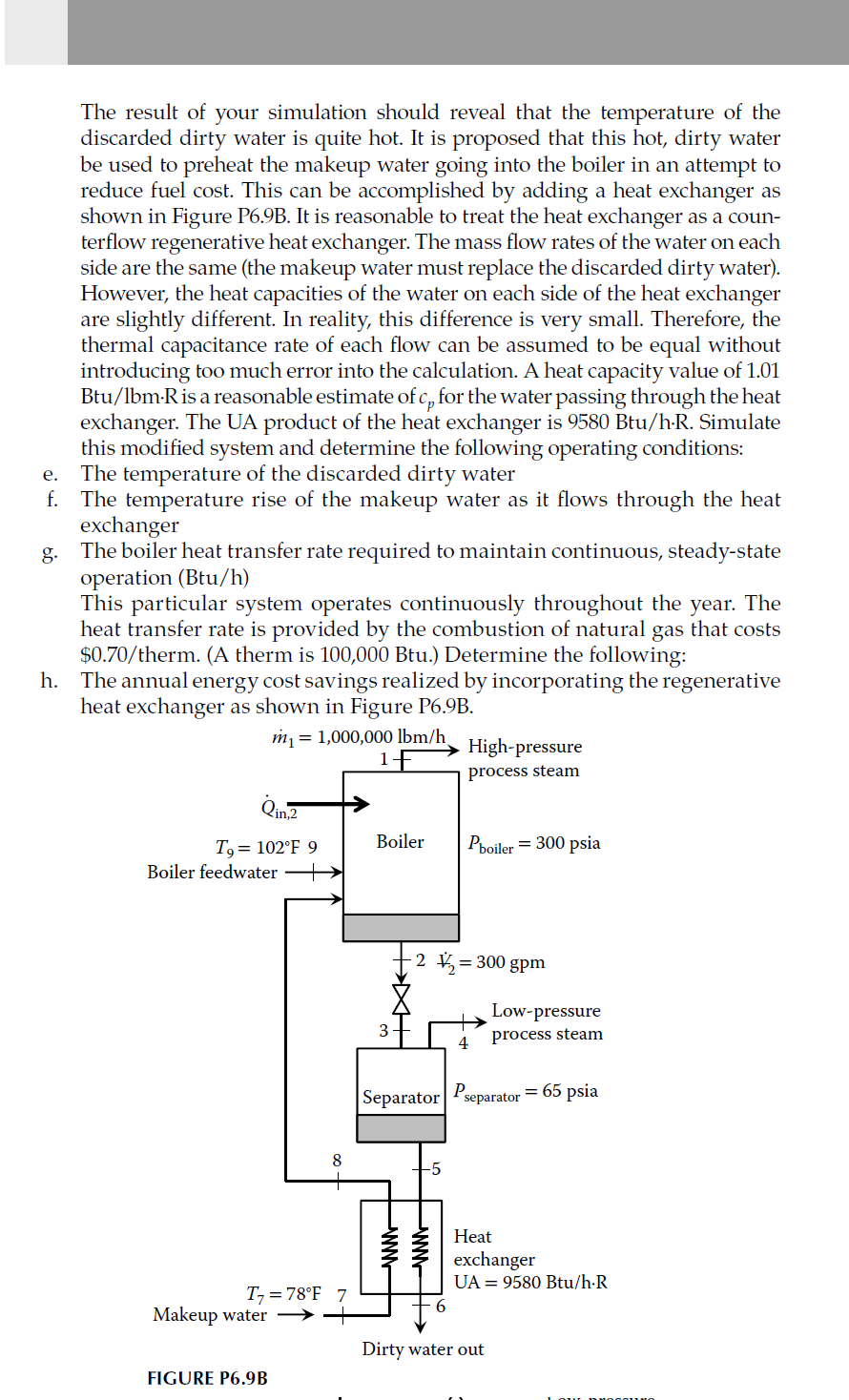
(Solved): can you show the solution for bits (e) to (h) of the problem statement? The result of your simulat ...

can you show the solution for bits (e) to (h) of the problem statement? The result of your simulation should reveal that the temperature of the discarded dirty water is quite hot. It is proposed that this hot, dirty water be used to preheat the makeup water going into the boiler in an attempt to reduce fuel cost. This can be accomplished by adding a heat exchanger as shown in Figure P6.9B. It is reasonable to treat the heat exchanger as a counterflow regenerative heat exchanger. The mass flow rates of the water on each side are the same (the makeup water must replace the discarded dirty water). However, the heat capacities of the water on each side of the heat exchanger are slightly different. In reality, this difference is very small. Therefore, the thermal capacitance rate of each flow can be assumed to be equal without introducing too much error into the calculation. A heat capacity value of 1.01 \( \mathrm{Btu} / \mathrm{lbm} \cdot \mathrm{R} \) is a reasonable estimate of \( c_{p} \) for the water passing through the heat exchanger. The UA product of the heat exchanger is \( 9580 \mathrm{Btu} / \mathrm{h} \cdot \mathrm{R} \). Simulate this modified system and determine the following operating conditions: e. The temperature of the discarded dirty water f. The temperature rise of the makeup water as it flows through the heat exchanger g. The boiler heat transfer rate required to maintain continuous, steady-state operation (Btu/h) This particular system operates continuously throughout the year. The heat transfer rate is provided by the combustion of natural gas that costs \( \$ 0.70 / \) therm. (A therm is 100,000 Btu.) Determine the following: h. The annual energy cost savings realized by incorporating the regenerative heat exchanger as shown in Figure P6.9B. FIGURE P6.9B